CP121 买股票的最佳时机

题目描述：

给定一个数组 prices ，它的第 i 个元素 prices[i] 表示一支给定股票第 i 天的价格。你只能选择 某一天 买入这只股票，并选择在 未来的某一个不同的日子 卖出该股票。设计一个算法来计算你所能获取的最大利润。返回你可以从这笔交易中获取的最大利润。如果你不能获取任何利润，返回 0 。

学习记录：

第一想法是双指针往中间走，但是存在问题，就是取到最大最小的地方我们不知道，无法判断指针的移动，这种方法不可行。还是老老实实用数组记录每一个下标处能获得的最大利润就行，然后找到这个数组的最大即可，可省略为一个存储空间，直接记录最大即可

//超简略一遍过！
class Solution {
public:int maxProfit(vector<int>& prices) {int min_value=prices[0];int result=0;for(int i=0;i<prices.size();i++){min_value=min(min_value,prices[i]);result=max(result,prices[i]-min_value);}return result;}
};

CP122 买股票的最佳时机II

题目描述：

给你一个整数数组 prices ，其中 prices[i] 表示某支股票第 i 天的价格。在每一天，你可以决定是否购买和/或出售股票。你在任何时候 最多 只能持有 一股 股票。你也可以先购买，然后在 同一天 出售。返回你能获得的最大利润 。

学习记录：

1.动态规划

想不出来转移函数...

 今天没有股票=昨天没有股票OR昨天有然后卖了；今天有=昨天有OR昨天没有今天买了；

//题解
class Solution {
public:int maxProfit(vector<int>& prices) {int n = prices.size();int dp[n][2];dp[0][0] = 0, dp[0][1] = -prices[0];for (int i = 1; i < n; ++i) {dp[i][0] = max(dp[i - 1][0], dp[i - 1][1] + prices[i]);dp[i][1] = max(dp[i - 1][1], dp[i - 1][0] - prices[i]);}return dp[n - 1][0];}
};

 简化一下

//题解
class Solution {
public:int maxProfit(vector<int>& prices) {int n = prices.size();int dp0 = 0, dp1 = -prices[0];for (int i = 1; i < n; ++i) {int newDp0 = max(dp0, dp1 + prices[i]);int newDp1 = max(dp1, dp0 - prices[i]);dp0 = newDp0;dp1 = newDp1;}return dp0;}
};

2.贪心法

其实我的思路应该是贪心法，没意识到，我想的是，就是只要能够获得利润，我就卖出，因为买入和卖出可以在同一天进行所以一定能获得更多的利润，但是不确定是不是最优

//题解
class Solution {
public:int maxProfit(vector<int>& prices) {   int ans = 0;int n = prices.size();for (int i = 1; i < n; ++i) {ans += max(0, prices[i] - prices[i - 1]);}return ans;}
};

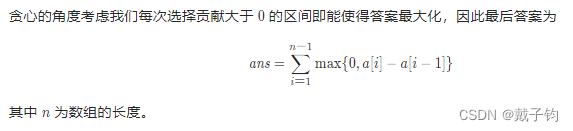
这里解决我刚才的疑惑，就是是否是最佳的。

CP123 买股票的最佳时机III

题目描述：

给定一个数组，它的第 i 个元素是一支给定的股票在第 i 天的价格。设计一个算法来计算你所能获取的最大利润。你最多可以完成两笔交易。注意：你不能同时参与多笔交易（你必须在再次购买前出售掉之前的股票）。

学习记录：

参考刚刚II的想法，然后没有思路....

 

//题解
class Solution {
public:int maxProfit(vector<int>& prices) {int n = prices.size();int buy1 = -prices[0], sell1 = 0;int buy2 = -prices[0], sell2 = 0;for (int i = 1; i < n; ++i) {buy1 = max(buy1, -prices[i]);sell1 = max(sell1, buy1 + prices[i]);buy2 = max(buy2, sell1 - prices[i]);sell2 = max(sell2, buy2 + prices[i]);}return sell2;}
};

CP188 买股票的最佳时机IV

题目描述：

给定一个整数数组 prices ，它的第 i 个元素 prices[i] 是一支给定的股票在第 i 天的价格，和一个整型 k 。设计一个算法来计算你所能获取的最大利润。你最多可以完成 k 笔交易。也就是说，你最多可以买 k 次，卖 k 次。注意：你不能同时参与多笔交易（你必须在再次购买前出售掉之前的股票）。

学习记录：

有思路和III一样的用n组selli和buyi来记录，其实刚刚III中的buy1，buy2，其实是buy[i]1，buy[i]2，也就是对应这里的buy[i][j]，因为之和前一天的有关系，就压缩到一个存储空间了。

 注意：

//题解
class Solution {
public:int maxProfit(int k, vector<int>& prices) {if (prices.empty()) {return 0;}int n = prices.size();k = min(k, n / 2);vector<vector<int>> buy(n, vector<int>(k + 1));vector<vector<int>> sell(n, vector<int>(k + 1));buy[0][0] = -prices[0];sell[0][0] = 0;for (int i = 1; i <= k; ++i) {buy[0][i] = sell[0][i] = INT_MIN / 2;}for (int i = 1; i < n; ++i) {buy[i][0] = max(buy[i - 1][0], sell[i - 1][0] - prices[i]);for (int j = 1; j <= k; ++j) {buy[i][j] = max(buy[i - 1][j], sell[i - 1][j] - prices[i]);sell[i][j] = max(sell[i - 1][j], buy[i - 1][j - 1] + prices[i]);   }}return *max_element(sell[n - 1].begin(), sell[n - 1].end());}
};

简化到一个存储空间：

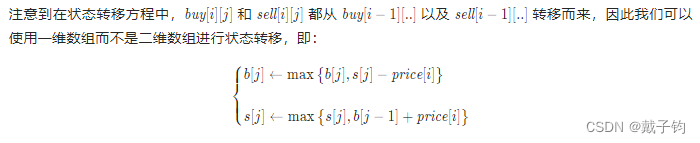
//题解
class Solution {
public:int maxProfit(int k, vector<int>& prices) {if (prices.empty()) {return 0;}int n = prices.size();k = min(k, n / 2);vector<int> buy(k + 1);vector<int> sell(k + 1);buy[0] = -prices[0];sell[0] = 0;for (int i = 1; i <= k; ++i) {buy[i] = sell[i] = INT_MIN / 2;}for (int i = 1; i < n; ++i) {buy[0] = max(buy[0], sell[0] - prices[i]);for (int j = 1; j <= k; ++j) {buy[j] = max(buy[j], sell[j] - prices[i]);sell[j] = max(sell[j], buy[j - 1] + prices[i]);   }}return *max_element(sell.begin(), sell.end());}
};

CP309 最佳买卖股票时机含冷冻期

题目描述：

给定一个整数数组prices，其中第  prices[i] 表示第 i 天的股票价格 。​设计一个算法计算出最大利润。在满足以下约束条件下，你可以尽可能地完成更多的交易（多次买卖一支股票）:卖出股票后，你无法在第二天买入股票 (即冷冻期为 1 天)。注意：你不能同时参与多笔交易（你必须在再次购买前出售掉之前的股票）。

学习记录：

一遍过，代码：

可以看到备注的情况，就是定义四种状态，sell，buy，nothing，cold，代表每天可能处于的情况，写出转移方程即可。

class Solution {
public:int maxProfit(vector<int>& prices) {int length=prices.size();if(length==1) return 0;int result;//buy:今天买 sell:今天卖 //nothing:今天有股票不做事情 cold:今天没股票但不做事情int buy=-prices[0],sell=0,nothing=-prices[0],cold=0;int temp;for(int i=1;i<length;i++){temp=sell;sell=max(max(buy,nothing)+prices[i],sell);nothing=max(buy,nothing);buy=cold-prices[i];cold=temp;}return sell;}
};

题解：可以看到题解定义的情况比我们少一种，是从当前手上有没有股票来进行定义的

class Solution {
public:int maxProfit(vector<int>& prices) {if (prices.empty()) {return 0;}int n = prices.size();// f[i][0]: 手上持有股票的最大收益// f[i][1]: 手上不持有股票，并且处于冷冻期中的累计最大收益// f[i][2]: 手上不持有股票，并且不在冷冻期中的累计最大收益vector<vector<int>> f(n, vector<int>(3));f[0][0] = -prices[0];for (int i = 1; i < n; ++i) {f[i][0] = max(f[i - 1][0], f[i - 1][2] - prices[i]);f[i][1] = f[i - 1][0] + prices[i];f[i][2] = max(f[i - 1][1], f[i - 1][2]);}return max(f[n - 1][1], f[n - 1][2]);}
};

PS：

就是很难想到转移方程，感觉转移方程有两种情况

1.逻辑关系，比如之前的走格子，你要么....要么....

2.如这几题的情况，考虑你的状态，你每次做完一个操作处在什么状态，每个状态之间是如何进行转变的，最后在哪个状态下取到你想要的结果