【数据结构】二叉搜索树BSTree

news/2025/1/3 3:18:54/

文章目录

    • 一、概念
    • 二、基础操作
        • 1.查找find
        • 2.插入Insert
        • 3.中序遍历InOrder
        • 4.删除erase
    • 三、递归写法
        • 1.递归查找
        • 2.递归插入
        • 3.递归删除
    • 四、应用
    • 五、题目练习

一、概念

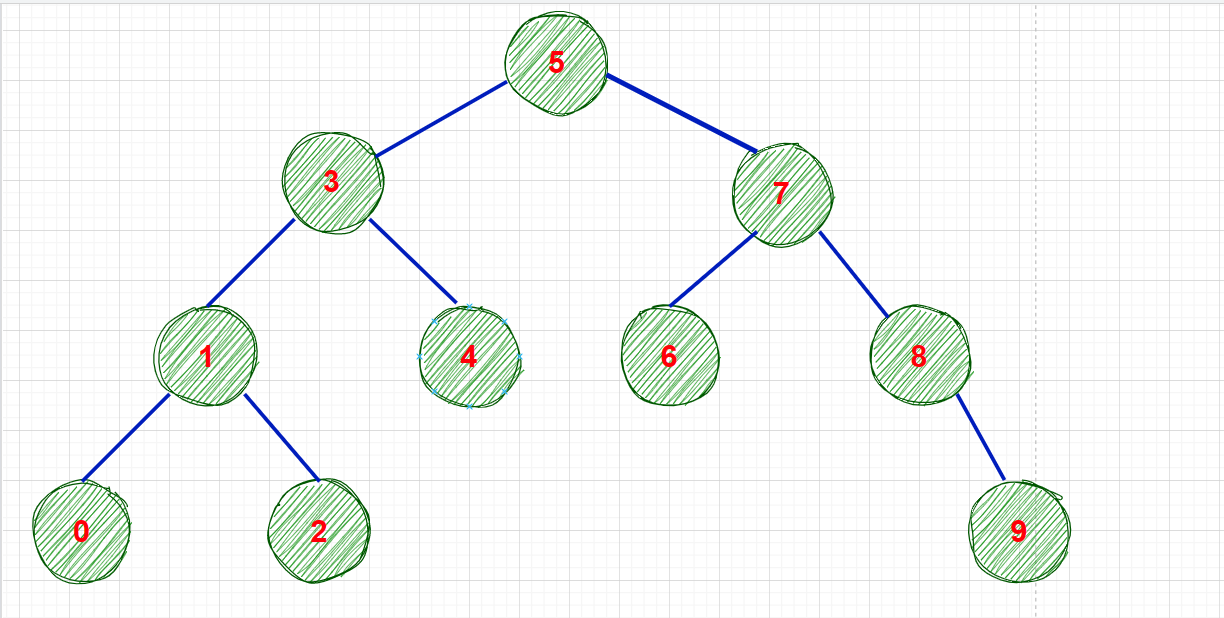
二叉搜索树又称二叉排序树，它或者是一棵空树，或者是具有以下性质的二叉树:

若它的左子树不为空，则左子树上所有节点的值都小于根节点的值

若它的右子树不为空，则右子树上所有节点的值都大于根节点的值

左<根<右

它的左右子树也分别为二叉搜索树

之所以又叫二叉排序树，是因为二叉搜索树中序遍历的结果是有序的

image-20230205153810150

二、基础操作

1.查找find

基于二叉搜索树的特点，查找一个数并不难，若根节点不为空的情况下：

若根节点key==查找key，直接返回true

若根节点key>查找key，那得找到更小的，则往左子树查找

若根节点key<查找key，那得找到更大的，则往右子树查找

最多查找高度次，走到空为止，如果还没找到，则说明这个值不存在，返回false

	bool find(const K& key){Node* cur = _root;while (cur){if (cur->_key < key){cur = cur->_right;}else if (cur->_key > key){cur = cur->_left;}else{return true;}}return false;}

2.插入Insert

1.树为空，则直接插入,新增节点，直接插入root指针即可

2.树不为空，按二叉搜索树性质查找插入位置，插入新节点。

（注意：不能插入重复的元素，并且每次插入都是要定位到空节点的位置；我们先定义一个 cur从root开始，比较元素的大小：若插入的元素比当前位置元素小就往左走，比当前位置元素大就往右走，直到为空,相等就不能插入了;同时定义一个parent去记录当前 cur的前一个位置，最后判断cur是parent的左子树还是右子树即可）

	bool Insert(const K& key){if (_root == nullptr){_root = new Node(key);return true;}Node* parent = nullptr;Node* cur = _root;while (cur){if (cur->_key < key){parent = cur;cur = cur->_right;}else if (cur->_key > key){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(key);if (parent->_key < key){parent->_right = cur;}else{parent->_left = cur;}return true;}

3.中序遍历InOrder

递归走起，同时由于_root是私有的，外部不能访问，我们可以在类内给中序提供一个方法即可，就不需要传参了

void InOrder(){_InOrder(_root);cout << endl;}private:void _InOrder(Node* root){if (root == nullptr){return;}_InOrder(root->_left);cout << root->_key << " ";_InOrder(root->_right);}Node* _root = nullptr;

4.删除erase

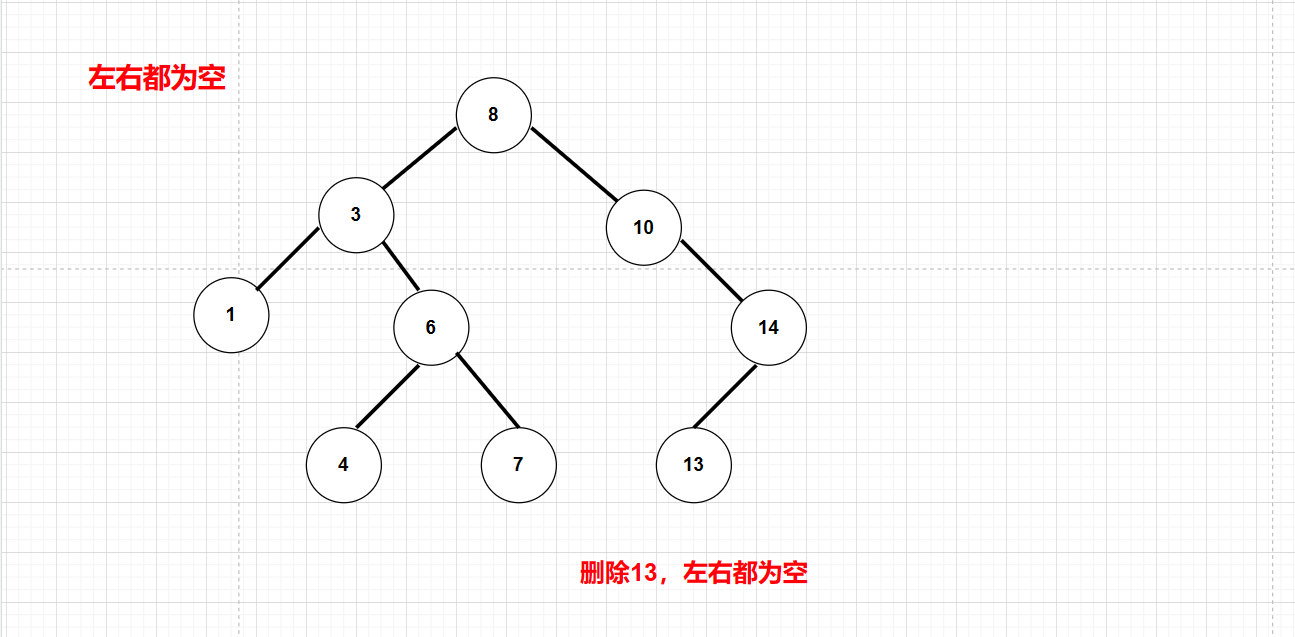
删除的情况比较多：

  1. 左右都为空：叶子结点，直接置空并链接到空指针
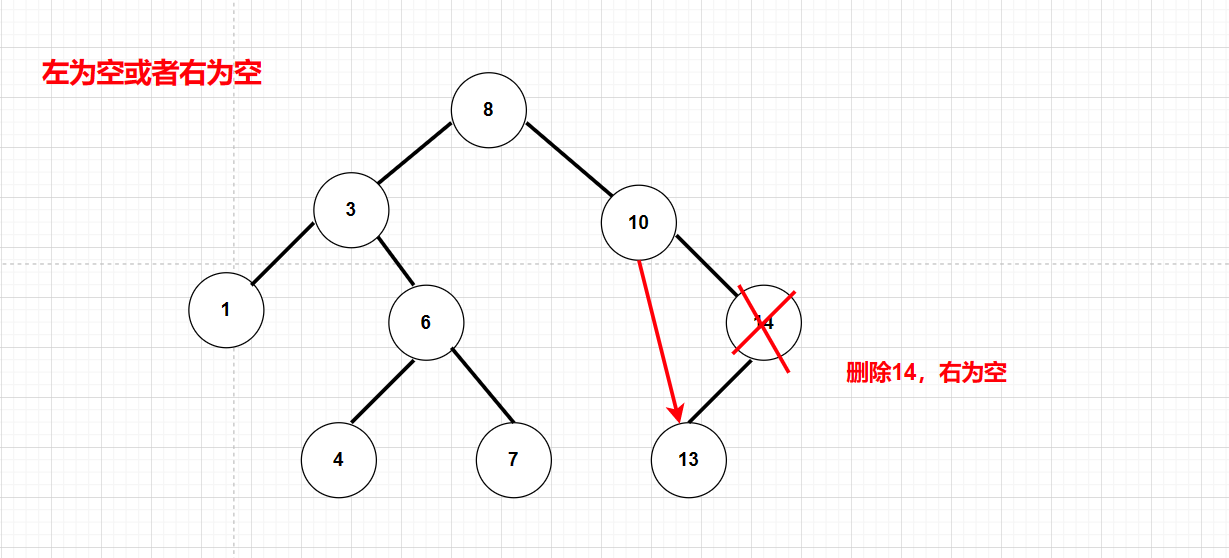
  2. 左为空或右为空：进行托孤：只有一个子节点，删除自己本身，并链接子节点和父节点（注意：如果父亲是空，也就是要删除根结点，此时根节点没有父亲，单独判断一下）
  3. 左右都不为空：找出替换节点：右子树最小节点**、**左子树最大节点。替换节点可以作为交换和删除进行交换，交换后删除交换节点、交换节点要么没有孩子，要么只有一个孩子可以直接删除

但是左右都为空可以纳入到左为空或右为空的情况

image-20230206100211916

image-20230206100420494

image-20230206102733973

注意：

image-20230206110838654

代码实现：

bool Erase(const K& key){Node* parent = nullptr;Node* cur = _root;while (cur){if (cur->_key < key){parent = cur;cur = cur->_right;}else if (cur->_key > key){parent = cur;cur = cur->_left;}else{//左为空if (cur->_left == nullptr){//删除根结点//if(parent==nullptr)if (cur == _root){_root = cur->_right;}else{if (parent->_left == cur){parent->_left = cur->_right;}else{parent->_right = cur->_right;}}delete cur;}//右为空else if (cur->_right == nullptr){if (cur == _root){_root = cur->_left;}else{if (parent->_left == cur){parent->_left = cur->_left;}else{parent->_left = cur->_left;}}delete cur;}//左右都不为空,找替换节点else{//不能初始化为nullptrNode* parent = cur;//右子树最小节点Node* minRight = cur->_right;while (minRight->_left){parent = minRight;minRight = minRight->_left;}cur->_key = minRight->_key;//判断minRight是父亲的左还是右if (minRight == parent->_left){parent->_left = minRight->_right;}else{parent->_right = minRight->_right;}delete minRight;}return true;}}return false;}

三、递归写法

1.递归查找

这个比较简单：苏醒把，递归时刻

bool _FindR(Node* root, const K& key){if (root == nullptr) return false;else if (root->_key < key) return _FindR(root->_right, key);else if (root->_key > key) return _FindR(root->_left, key);else return true;}

2.递归插入

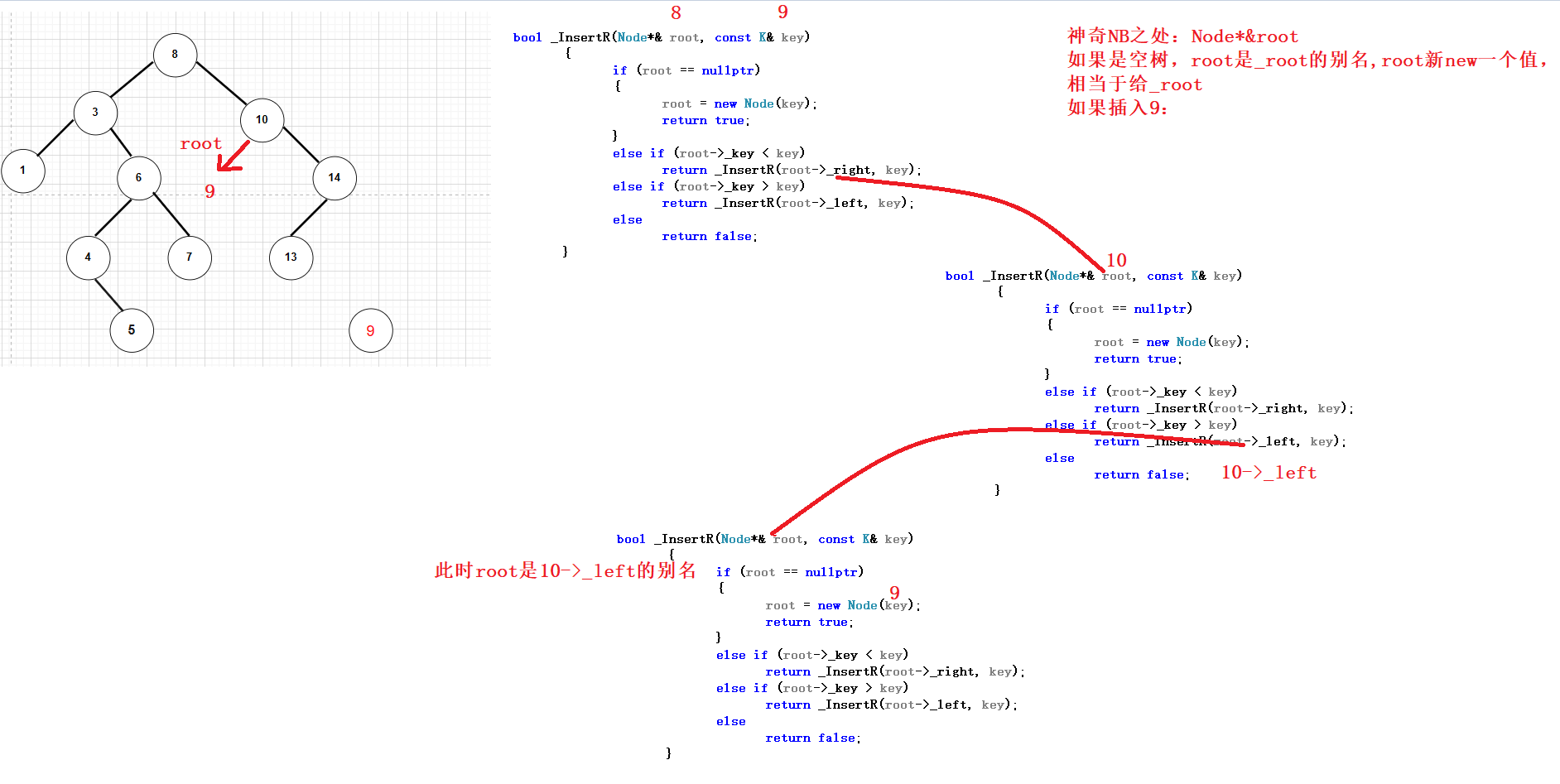
最大的问题是插入之后跟父亲进行链接，如果直接给root是不可以的，因为root是栈帧里面的参数，只是局部变量：加上引用

bool _InsertR(Node*& root, const K& key){if (root == nullptr){root = new Node(key);return true;}else if (root->_key < key) return _InsertR(root->_right, key);else if (root->_key > key) return _InsertR(root->_left, key);else return false;}

image-20230206123108961

3.递归删除

递归删除怎么找到父节点？root = root->_left/ root = root->_right;

image-20230206144325890

bool _EraseR(Node*& root, const K& key){if (root == nullptr){return false;}if (root->_key < key){return _EraseR(root->_right, key);}else if (root->_key > key){return _EraseR(root->_left, key);}else{Node* del = root;if (root->_right == nullptr){root = root->_left;}else if (root->_left == nullptr){root = root->_right;}else{Node* minRight = root->_right;while (minRight->_left){minRight = minRight->_left;}swap(root->_key, minRight->_key);return _EraseR(root->_right, key);}delete del;return true;}}

四、应用

最优情况下，二叉搜索树为完全二叉树，其平均比较次数为：log2N
最差情况下，二叉搜索树退化为单支树，其平均比较次数为： N/2

image-20230207171334088

1.K模型：K模型即只有key作为关键码，结构中只需要存储Key即可，关键码即为需要搜索到的值，判断关键字是否存在。
比如：给一个单词word，判断该单词是否拼写正确，具体方式如下：
以单词集合中的每个单词作为key，构建一棵二叉搜索树，在二叉搜索树中检索该单词是否存在，存在则拼写正确，不存在则拼写错误。

2.KV模型：每一个关键码key，都有与之对应的值Value，即**<Key, Value>**的键值对。

比如英汉词典就是英文与中文的对应关系，通过英文可以快速找到与其对应的中文，英文单词与其对应的中文<word, chinese>就构成一种键值对；再比如统计单词次数，统计成功后，给定单词就可快速找到其出现的次数，单词与其出现次数就是**<word, count>**就构成一种键值对。

namespace KV
{template <class K,class V>struct BSTreeNode{BSTreeNode<K,V>* _left;BSTreeNode<K,V>* _right;K _key;V _value;BSTreeNode(const K& key,const V&value):_key(key),_value(value),_left(nullptr),_right(nullptr){}};template <class K,class V>class BSTree{typedef BSTreeNode<K, V> Node;public:bool Insert(const K& key, const V& value)Node* find(const K& key)void InOrder()private:Node* _root = nullptr;};
}
void TestBSTree()
{//key/Value的搜索模型；通过key查找或修改ValueKV::BSTree<string, string> dict;dict.Insert("sort", "排序");dict.Insert("string", "字符串");dict.Insert("left", "左");dict.Insert("right", "右");string str;while (cin >> str){KV::BSTreeNode<string, string>* ret = dict.find(str);if (ret){cout << ret->_value << endl;}else{cout << "找不到" << endl;}}}

源代码：

BSTree.h

#include <iostream>
using namespace std;namespace K
{template <class K>struct BSTreeNode{BSTreeNode<K>* _left;BSTreeNode<K>* _right;K _key;BSTreeNode(const K& key):_key(key),_left(nullptr),_right(nullptr){}};template <class K>class BSTree{typedef BSTreeNode<K> Node;public:BSTree():_root(nullptr){}BSTree(const BSTree<K>& t){_root = Copy(t._root);}BSTree<K>& operator = (BSTree<K> t){swap(_root, t._root);return *this;}~BSTree(){Destroy(_root);_root = nullptr;}bool Insert(const K& key){if (_root == nullptr){_root = new Node(key);return true;}Node* parent = nullptr;Node* cur = _root;while (cur){if (cur->_key < key){parent = cur;cur = cur->_right;}else if (cur->_key > key){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(key);if (parent->_key < key){parent->_right = cur;}else{parent->_left = cur;}return true;}bool find(const K& key){Node* cur = _root;while (cur){if (cur->_key < key){cur = cur->_right;}else if (cur->_key > key){cur = cur->_left;}else{return true;}}return false;}bool Erase(const K& key){Node* parent = nullptr;Node* cur = _root;while (cur){if (cur->_key < key){parent = cur;cur = cur->_right;}else if (cur->_key > key){parent = cur;cur = cur->_left;}else{//左为空if (cur->_left == nullptr){//删除根结点//if(parent==nullptr)if (cur == _root){_root = cur->_right;}else{if (parent->_left == cur){parent->_left = cur->_right;}else{parent->_right = cur->_right;}}delete cur;}//右为空else if (cur->_right == nullptr){if (cur == _root){_root = cur->_left;}else{if (parent->_left == cur){parent->_left = cur->_left;}else{parent->_left = cur->_left;}}delete cur;}//左右都不为空,找替换节点else{//不能初始化为nullptrNode* parent = cur;//右子树最小节点Node* minRight = cur->_right;while (minRight->_left){parent = minRight;minRight = minRight->_left;}cur->_key = minRight->_key;//判断minRight是父亲的左还是右if (minRight == parent->_left){parent->_left = minRight->_right;}else{parent->_right = minRight->_right;}delete minRight;}return true;}}return false;}void InOrder(){_InOrder(_root);cout << endl;}//递归bool InsertR(const K& key){return _InsertR(_root, key);}bool FindR(const K& key){return _FindR(_root, key);}bool EraseR(const K& key){return _EraseR(_root, key);}private:void Destroy(Node* root){if (root == nullptr){return;}Destroy(root->_left);Destroy(root->_right);delete root;}Node* Copy(Node* root){if (root == nullptr)return nullptr;Node* newRoot = new Node(root->_key);newRoot->_left = Copy(root->_left);newRoot->_right = Copy(root->_right);return newRoot;}bool _EraseR(Node*& root, const K& key){if (root == nullptr){return false;}if (root->_key < key){return _EraseR(root->_right, key);}else if (root->_key > key){return _EraseR(root->_left, key);}else{Node* del = root;if (root->_right == nullptr){root = root->_left;}else if (root->_left == nullptr){root = root->_right;}else{Node* minRight = root->_right;while (minRight->_left){minRight = minRight->_left;}swap(root->_key, minRight->_key);return _EraseR(root->_right, key);}delete del;return true;}}bool _InsertR(Node*& root, const K& key){if (root == nullptr){root = new Node(key);return true;}else if (root->_key < key)return _InsertR(root->_right, key);else if (root->_key > key)return _InsertR(root->_left, key);elsereturn false;}bool _FindR(Node* root, const K& key){if (root == nullptr) return false;else if (root->_key < key) return _FindR(root->_right, key);else if (root->_key > key) return _FindR(root->_left, key);else return true;}void _InOrder(Node* root){if (root == nullptr){return;}_InOrder(root->_left);cout << root->_key << " ";_InOrder(root->_right);}Node* _root = nullptr;};
}namespace KV
{template <class K,class V>struct BSTreeNode{BSTreeNode<K,V>* _left;BSTreeNode<K,V>* _right;K _key;V _value;BSTreeNode(const K& key,const V&value):_key(key),_value(value),_left(nullptr),_right(nullptr){}};template <class K,class V>class BSTree{typedef BSTreeNode<K, V> Node;public:bool Insert(const K& key, const V& value){if (_root == nullptr){_root = new Node(key, value);return true;}Node* parent = nullptr;Node* cur = _root;while (cur){if (cur->_key < key){parent = cur;cur = cur->_right;}else if (cur->_key > key){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(key, value);if (parent->_key < key){parent->_right = cur;}else{parent->_left = cur;}return true;}Node* find(const K& key){Node* cur = _root;while (cur){if (cur->_key < key){cur = cur->_right;}else if (cur->_key > key){cur = cur->_left;}else{return cur;}}return nullptr;}void InOrder(){_InOrder(_root);}private:void _InOrder(Node* root){if (root == nullptr) return;_InOrder(root->_left);cout << root->_key << ":"<<root->_value<<endl;_InOrder(root->_right);}Node* _root = nullptr;};
}
void TestBSTree1()
{int a[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };K::BSTree<int> t;for (auto e : a){t.Insert(e);}t.InOrder();K::BSTree<int> copyt(t);copyt.InOrder();t.InsertR(9);t.InOrder();t.EraseR(9);t.InOrder();t.EraseR(3);t.InOrder();for (auto e : a){t.EraseR(e);t.InOrder();}
}
void TestBSTree2()
{KV::BSTree<string, string> dict;dict.Insert("sort", "排序");dict.Insert("string", "字符串");dict.Insert("left", "左");dict.Insert("right", "右");string str;while (cin >> str){KV::BSTreeNode<string, string>* ret = dict.find(str);if (ret){cout << ret->_value << endl;}else{cout << "找不到" << endl;}}}
void TestBSTree3()
{string arr[] = { "苹果","西瓜","苹果" };KV::BSTree<string, int> countTree;for (auto e : arr){auto* ret = countTree.find(e);if (ret == nullptr){countTree.Insert(e, 1);}else{ret->_value++;}}countTree.InOrder();
}

#include "BSTree.h"
int main()
{//TestBSTree1();TestBSTree2();//TestBSTree3();return 0;
}

五、题目练习

根据二叉树创建字符串

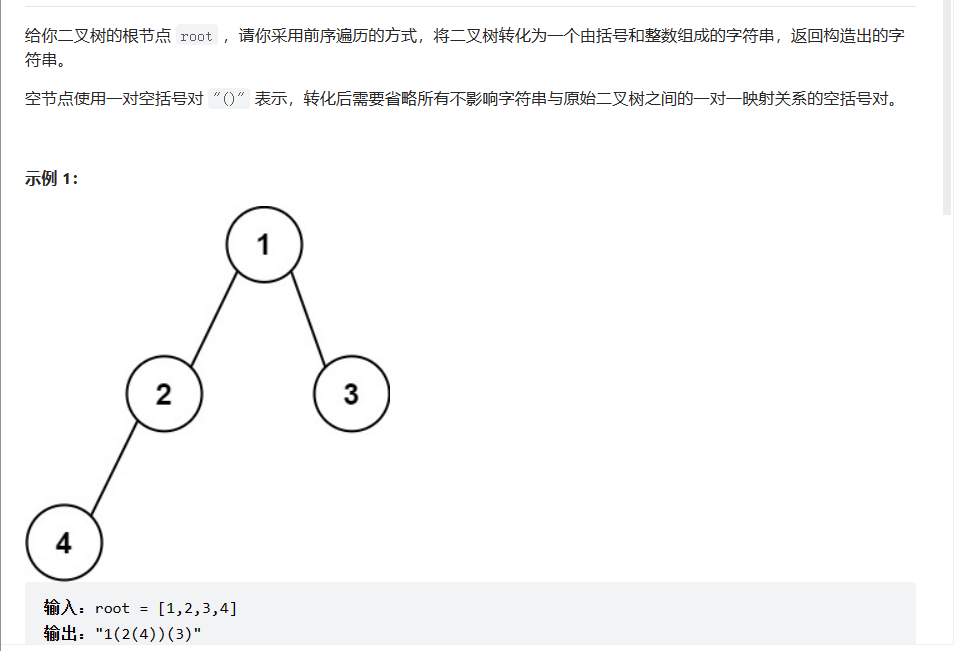
image-20230207135712593

前序遍历，左为空，右不为空的括号不可以省略，右为空的括号可以省略

class Solution {
public:string tree2str(TreeNode* root) {if(root == nullptr) return string();string ret;ret += to_string(root->val);if(root->left){ret+='(';ret+= tree2str(root->left);ret+=')';}else if(root->right){ret+="()";}if(root->right){ret+='(';ret+=tree2str(root->right);ret+=')';}return ret;}
};

二叉树的层序遍历

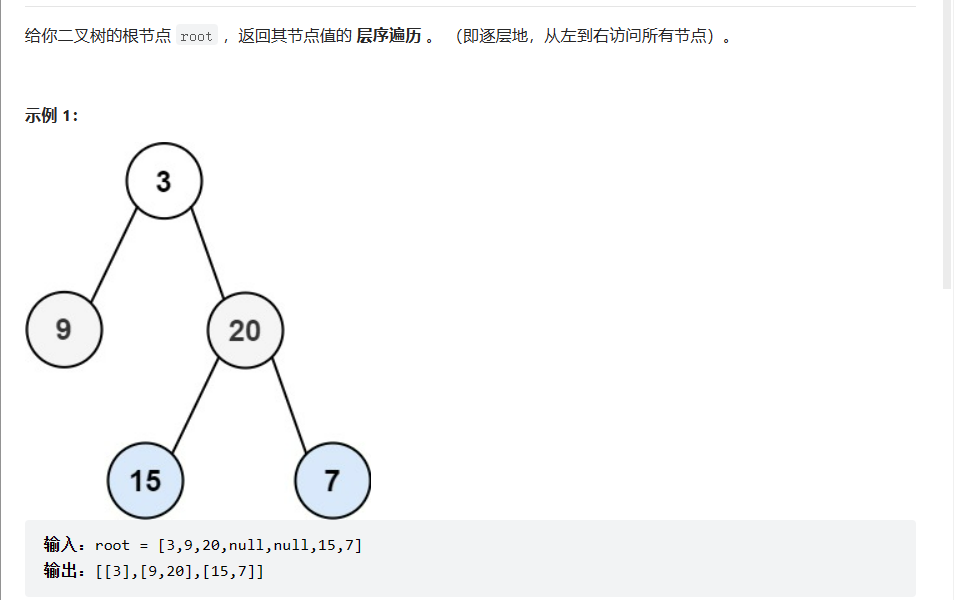
image-20230207140116868

层序遍历，可以通过一个队列来实现，同时定义每次队列的大小

class Solution {
public:vector<vector<int>> levelOrder(TreeNode* root) {queue<TreeNode*> q;vector<vector<int>> vv;size_t levelSize = 0;if(root){q.push(root);levelSize=1;}while(!q.empty()){vector<int> v;while(levelSize--){TreeNode* front = q.front();q.pop();v.push_back(front->val);if(front->left){q.push(front->left);}if(front->right){q.push(front->right);}}vv.push_back(v);levelSize = q.size();}return vv;}
};

二叉树的最近公共祖先

image-20230207141239596

image-20230207142901630

class Solution {bool isInTree(TreeNode*root,TreeNode*x){if(root == nullptr) return false;if(root == x) return true;else return isInTree(root->left,x)|| isInTree(root->right,x);}
public:TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {if(root==nullptr)return nullptr;if(root == p||root==q) return root;bool pLeft = isInTree(root->left,p);bool pRight = !pLeft;bool qLeft = isInTree(root->left,q);bool qRight = !qLeft;//一个在左一个在右if((pLeft&&qRight)||(pRight&&qLeft))return root;//同左if(pLeft&&qLeft)return lowestCommonAncestor(root->left,p,q);//同右elsereturn lowestCommonAncestor(root->right,p,q);}
};

把根到对应节点的路径存储起来，在找出相交的结点即是最近的公共结点：

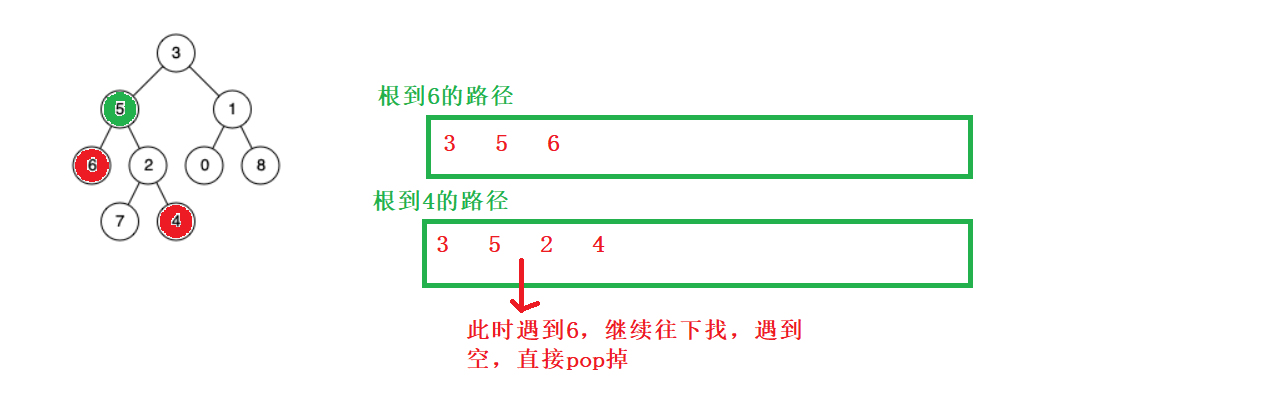
image-20230207151621173

class Solution {bool GetPath(TreeNode*root,TreeNode*x,stack<TreeNode*>& stack){if(root == nullptr) return false;stack.push(root);if(root == x){return true;}if(GetPath(root->left,x,stack))return true;if(GetPath(root->right,x,stack))return true;stack.pop();return false;}
public:TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {if(root==nullptr)return nullptr;stack<TreeNode*> pPath;stack<TreeNode*> qPath;GetPath(root,p,pPath);GetPath(root,q,qPath);//长的先popwhile(pPath.size()!=qPath.size()){if(pPath.size()>qPath.size()){pPath.pop();}elseqPath.pop();}//同时pop,找出交点while(pPath.top()!=qPath.top()){pPath.pop();qPath.pop();}return pPath.top();}
};

二叉搜索树与双向链表

image-20230207132853903

思路一：中序遍历，将节点放到一个vector中，在链接节点，但是空间复杂度不符合题目要求：

class Solution {void InOrder(TreeNode*root,vector<TreeNode*>& v){if(root==nullptr) return;InOrder(root->left,v);v.push_back(root);InOrder(root->right,v);}
public:TreeNode* Convert(TreeNode* pRootOfTree) {if(pRootOfTree==nullptr) return nullptr;vector<TreeNode*> v;InOrder(pRootOfTree,v);if(v.size()<=1) return v[0];v[0]->left =nullptr;v[0]->right = v[1];for(int i =1;i<v.size()-1;i++){v[i]->left = v[i-1];v[i]->right = v[i+1];}v[v.size()-1]->left = v[v.size()-2];v[v.size()-1]->right = nullptr;return v[0];}
};

思路二：递归直接进行转换

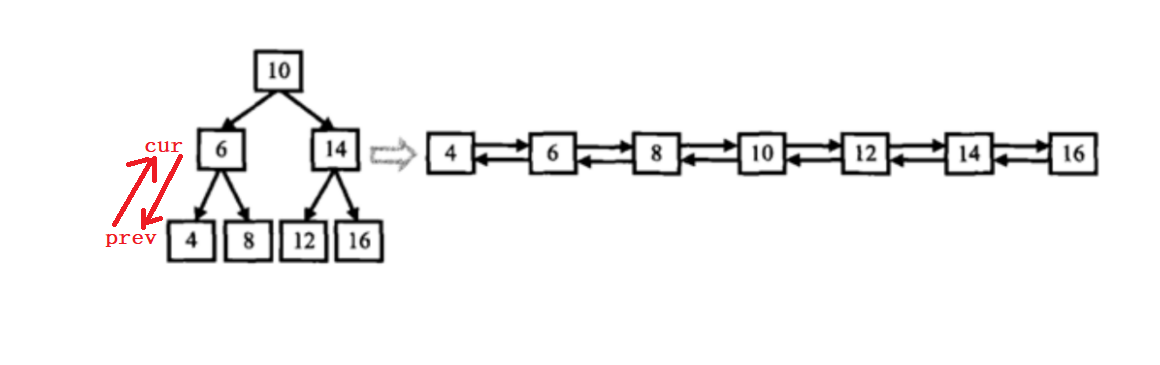
image-20230207134742988

class Solution {void InOrder(TreeNode*cur,TreeNode*&prev){if(cur==nullptr){return;}InOrder(cur->left,prev);cur->left = prev;if(prev){prev->right = cur;}prev = cur;InOrder(cur->right,prev);}public:TreeNode* Convert(TreeNode* pRootOfTree) {TreeNode*prev = nullptr;InOrder(pRootOfTree,prev);//找头TreeNode*head = pRootOfTree;while(head&&head->left){head = head->left;}return head;}
};

从前序与中序遍历序列构造二叉树

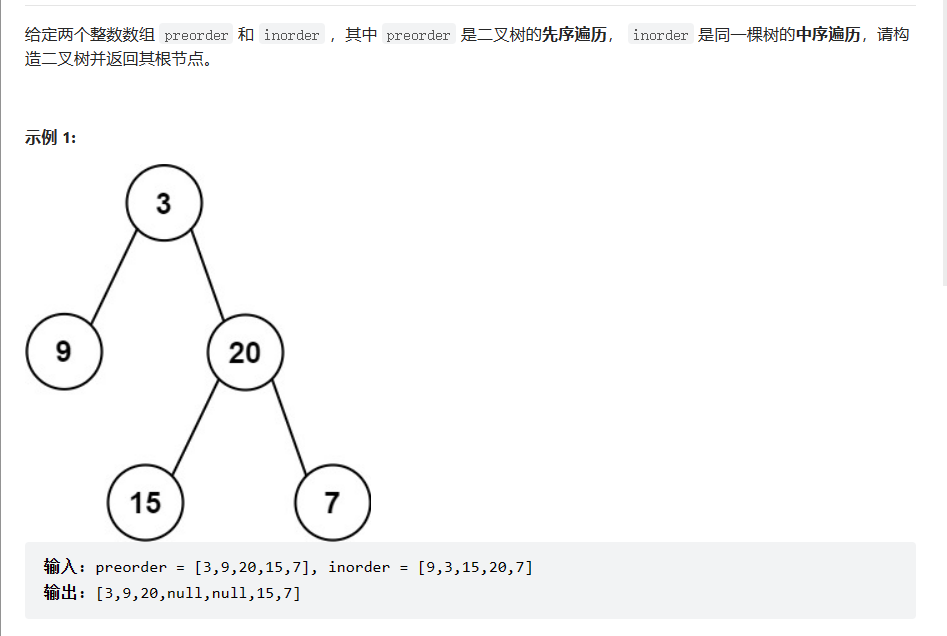
image-20230208124513337

根据前序结果去创建树，前序是根左右，前序第一个元素就是根，在通过中序去进行分割左右子树。子树区间确认是否继续递归创建子树，区间不存在则是空树。所以根据前序先构造根，在通过中序构造左子树、在构造右子树即可。

class Solution {TreeNode* _buildTree(vector<int>& preorder, vector<int>& inorder,int&prei,int inbegin,int inend){if(inbegin>inend){return nullptr;}TreeNode*root = new TreeNode(preorder[prei]);int rooti = inbegin;while(inbegin<=inend){if(preorder[prei] == inorder[rooti]){break;}else rooti++;}prei++;//[inbegin,rooti-1]rooti[rooti+1,inend]root->left= _buildTree(preorder,inorder,prei,inbegin,rooti-1);root->right = _buildTree(preorder,inorder,prei,rooti+1,inend);return root;}
public:TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {int prei = 0;return _buildTree(preorder,inorder,prei,0,inorder.size()-1);}
};

传引用问题：因为prei是遍历前序数组开始的下标，整个递归遍历中都要使用，所以我们需要传引用。如果不是传引用而是传值的话，左子树构建好返回，如果此时prei不是传引用，只是形参，无法将上一次递归的结果保留下来，那么也就无构建右子树了。

从中序与后序遍历序列构造二叉树

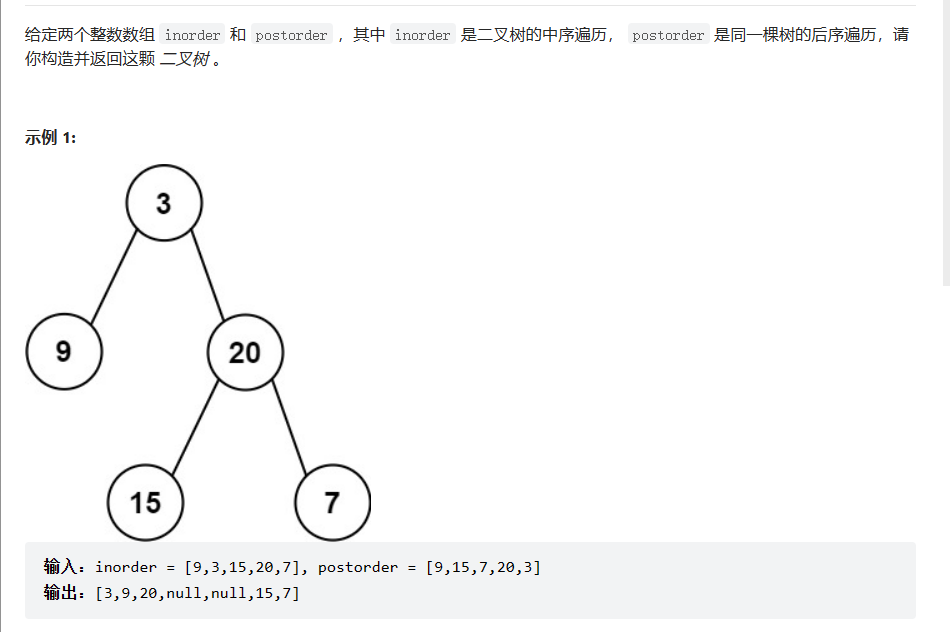
image-20230207162636352

根据后序遍历的最后一个元素可以确定根结点，有了根结点做为切割点然后再去根据中序遍历划分左右区间，在继续下去，构造成二叉树，区间不存在就是空树了。同时，后序遍历是左右根，所以最后一个是根节点。所以当我们构造根结点后，由于前面是右子树，所以先构造右子树，在构造左子数。

class Solution {TreeNode* _buildTree(vector<int>& inorder, vector<int>& postorder,int &posi,int inbegin,int inend){if(inbegin>inend){return nullptr;}TreeNode* root = new TreeNode(postorder[posi]);int rooti = inbegin;while(inbegin<=inend){if(postorder[posi] == inorder[rooti]){break;}else rooti++;}posi--;//[inbegin,rooti-1]rooti[rooti+1,inend];root->right = _buildTree(inorder,postorder,posi,rooti+1,inend);root->left = _buildTree(inorder,postorder,posi,inbegin,rooti-1);return root;}
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {int posi = postorder.size()-1;return _buildTree(inorder,postorder,posi,0,inorder.size()-1);}
};

image-20230208124414901


http://www.ppmy.cn/news/23321.html

相关文章

thinkphp关于模型一对多，多对多，多对一的使用

thinkphp关于模型一对多，多对多，多对一的使用

首先看看thinkphp5.0的模型介绍这里关联有一对一&#xff0c;多对多&#xff0c;一对多&#xff0c;多对一。还有预载入&#xff08;就是查询预写好&#xff0c;php界面调用时候才查询&#xff09;还有关联统计&#xff0c;聚合等。这里只讲解通过一对一的预载入&#xff0c;推…
阅读更多...
Day19 C++STL入门基础知识十一——map、multimap容器 构造赋值、大小交换、插入删除、查找统计、排序【全面深度剖析+例题代码展示】

Day19 C++STL入门基础知识十一——map、multimap容器 构造赋值、大小交换、插入删除、查找统计、排序【全面深度剖析+例题代码展示】

&#x1f483;&#x1f3fc; 本人简介&#xff1a;男 &#x1f476;&#x1f3fc; 年龄&#xff1a;18 ✍每日一句&#xff1a;【道固远&#xff0c;笃行可至&#xff1b;事虽巨&#xff0c;坚为必成】 文章目录1. 基本概念2. 构造赋值① 函数原型② 代码展示③ 测试结果3. 大小…
阅读更多...
初次认识C++类

初次认识C++类

目录 前言&#xff1a; 面向过程和面向对象的区别&#xff1a; C语言&#xff1a; C&#xff1a; 类的引入&#xff1a; 类的定义&#xff1a; 类的权限&#xff1a; 类的作用域&#xff1a; 类的实例化&#xff1a; 类的大小计算&#xff1a; 空类或则只…
阅读更多...
前端基础html css js

前端基础html css js

html&#xff1a;框架 css&#xff1a;美化 jp&#xff1a;交互 HTML 1.基础标签 <!DOCTYPE html> <html><head><meta charset"UTF-8"><title>这是我的滴滴滴一个网页</title></head><body><h1>一号 标题&…
阅读更多...
ROS2机器人编程简述humble-第四章-The TF Subsystem

ROS2机器人编程简述humble-第四章-The TF Subsystem

这个是ROS2机器人的核心工具之一。概念&#xff1a;TF&#xff08;坐标系转换&#xff09;子系统是ROS2机器人框架中的一个重要组件&#xff0c;它的功能是提供坐标系转换服务&#xff0c;使得不同坐标系之间的数据可以转换。比如&#xff0c;机器人的传感器可以产生的数据是基…
阅读更多...
【JavaEE】Java中复杂的Synchronized关键字

【JavaEE】Java中复杂的Synchronized关键字

目录 一、synchronized的特性 &#xff08;1&#xff09;互斥 &#xff08;2&#xff09;刷新内存 &#xff08;3&#xff09;可重入 二、synchronized的使用 &#xff08;1&#xff09;修饰普通方法 &#xff08;2&#xff09;修饰静态方法 &#xff08;3&#xff09;修…
阅读更多...
JDK8 新特性详解，2014-03-18 正式发布

JDK8 新特性详解，2014-03-18 正式发布

简介&#xff1a;JDK8 的主要新特性六个&#xff1a;Lambda、Stream、Date、新注解、函数编程、并发&#xff0c;前两者主要用于集合中。 1、Lambda 演变过程 Data ToString NoArgsConstructor AllArgsConstructor public class Student { //名字 private String name; //性别…
阅读更多...
OpenFST、WFST 小记

OpenFST、WFST 小记

文章目录关于 OpenFST安装 openfst关于 WFST编译 WFST关于 OpenFST 官网&#xff1a;https://www.openfst.org/twiki/bin/view/FST/WebHome快速入门文档&#xff1a;https://www.openfst.org/twiki/bin/view/FST/FstQuickTour下载&#xff1a;https://www.openfst.org/twiki/b…
阅读更多...
最新文章

Copyright @ 2022~2023