文章目录
- Orthogonal complements
- d i m ( V ) + d i m ( V ⊥ ) = n dim(V)+dim(V^\perp)=n dim(V)+dim(V⊥)=n
- Representing vectors in R n R^n Rn using subspace members
- ( V ⊥ ) ⊥ (V^\perp)^\perp (V⊥)⊥
- Unique rowspace solution to A x = b Ax = b Ax=b
- Rowspace solution to Ax = b example
Orthogonal complements
orthogonal complement 正交补 V ⊥ V^\perp V⊥
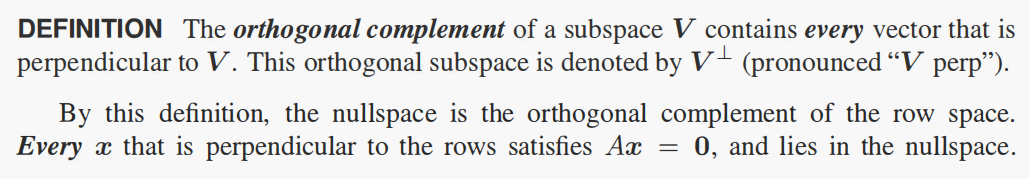
V ⊥ V^\perp V⊥ is a subspace.

N ( A ) = ( R ( A ) ) ⊥ N(A)=(R(A))^\perp N(A)=(R(A))⊥

N ( B T ) = ( C ( B ) ) ⊥ N(B^T)=(C(B))^\perp N(BT)=(C(B))⊥

d i m ( V ) + d i m ( V ⊥ ) = n dim(V)+dim(V^\perp)=n dim(V)+dim(V⊥)=n
V V V is a subspace of R n R^n Rn. 
Representing vectors in R n R^n Rn using subspace members





( V ⊥ ) ⊥ (V^\perp)^\perp (V⊥)⊥



Unique rowspace solution to A x = b Ax = b Ax=b



Rowspace solution to Ax = b example





