题目:
Minimal Ratio Tree
Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 1140 Accepted Submission(s): 348
Problem Description
For a tree, which nodes and edges are all weighted, the ratio of it is calculated according to the following equation.
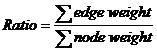
Given a complete graph of n nodes with all nodes and edges weighted, your task is to find a tree, which is a sub-graph of the original graph, with m nodes and whose ratio is the smallest among all the trees of m nodes in the graph.
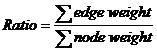
Given a complete graph of n nodes with all nodes and edges weighted, your task is to find a tree, which is a sub-graph of the original graph, with m nodes and whose ratio is the smallest among all the trees of m nodes in the graph.
Input
Input contains multiple test cases. The first line of each test case contains two integers n (2<=n<=15) and m (2<=m<=n), which stands for the number of nodes in the graph and the number of nodes in the minimal ratio tree. Two zeros end the input. The next line contains n numbers which stand for the weight of each node. The following n lines contain a diagonally symmetrical n×n connectivity matrix with each element shows the weight of the edge connecting one node with another. Of course, the diagonal will be all 0, since there is no edge connecting a node with itself.
All the weights of both nodes and edges (except for the ones on the diagonal of the matrix) are integers and in the range of [1, 100].
The figure below illustrates the first test case in sample input. Node 1 and Node 3 form the minimal ratio tree.
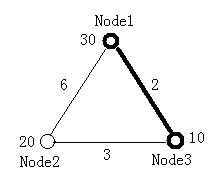
All the weights of both nodes and edges (except for the ones on the diagonal of the matrix) are integers and in the range of [1, 100].
The figure below illustrates the first test case in sample input. Node 1 and Node 3 form the minimal ratio tree.

Output
For each test case output one line contains a sequence of the m nodes which constructs the minimal ratio tree. Nodes should be arranged in ascending order. If there are several such sequences, pick the one which has the smallest node number; if there's a tie, look at the second smallest node number, etc. Please note that the nodes are numbered from 1 .
Sample Input
3 2 30 20 10 0 6 2 6 0 3 2 3 0 2 2 1 1 0 2 2 0 0 0
Sample Output
1 3 1 2
Source
2008 Asia Regional Beijing
Recommend
gaojie
深搜枚举m个点的子图,精度问题害死人。。 判小于的时候要判相减<-(1e-9) 才能对。。
//problem_id hdoj 2489
#include<set>
#include<map>
#include<cmath>
#include<queue>
#include<stack>
#include<vector>
#include<cstdio>
#include<cstring>
#include<iostream>
#include<algorithm>
using namespace std;
#define INF 0x1f1f1f1f
#define MIN(a,b) ((a)<(b)?(a):(b))
#define MAX(a,b) ((a)>(b)?(a):(b))
int edge_wei[20][20];//边权
int node_wei[20];//点权
bool flag[20];
bool fl[20];
double res;
int dfs_cnt;
bool arr[20];//result array
int n,m;
int mst()
{
int ret=0;
int low[20]={0};
int sta;
for(int i=1;i<=n;i++)
{
if(fl[i])
{
sta=i;
break;
}
}
low[sta]=0;
flag[sta]=1;
for(int i=1;i<=n;i++)
{
if(fl[i])
{
low[i]=edge_wei[sta][i];
}
}
for(int i=1;i<m;i++)
{
int min=INF;
int loc;
for(int j=1;j<=n;j++)
{
if(fl[j]&&!flag[j]&&low[j]<min)
{
min=low[j];
loc=j;
}
}
flag[loc]=1;
ret+=low[loc];
for(int j=1;j<=n;j++)
{
if(fl[j]&&!flag[j])
{
if(edge_wei[loc][j]<low[j])
{
low[j]=edge_wei[loc][j];
}
}
}
}
return ret;
}
void dfs(int v)
{
fl[v]=1;
dfs_cnt++;
if(dfs_cnt==m)
{
memset(flag,0,sizeof(flag));
int r=mst();
int sum=0;
for(int i=1;i<=n;i++)
{
if(fl[i])
{
sum+=node_wei[i];
}
}
double res_tmp=(double)r/(double)sum;
if(res_tmp-res<-(1e-9))//精度~~~
{
res=res_tmp;
for(int i=1;i<=n;i++)
{
arr[i]=fl[i];
}
}
fl[v]=0;
dfs_cnt--;
return;
}
for(int i=v+1;i<=n;i++)
{
dfs(i);
}
fl[v]=0;
dfs_cnt--;
}
int main()
{
while(scanf("%d%d",&n,&m),n||m)
{
for(int i=1;i<=n;i++)
{
scanf("%d",&node_wei[i]);
}
for(int i=1;i<=n;i++)
{
for(int j=1;j<=n;j++)
{
scanf("%d",&edge_wei[i][j]);
}
}
res=(double)INF;
for(int i=1;i<=n-m+1;i++)
{
memset(fl,0,sizeof(fl));
dfs_cnt=0;
dfs(i);
}
int fir=1;
for(int i=1;i<=n;i++)
{
if(arr[i])
{
if(fir)
{
fir=0;
printf("%d",i);
}
else
{
printf(" %d",i);
}
}
}
printf("\n");
}
return 0;
}